Skip to main content
Boundary Value Problems
Download PDF

The Monotone Iterative Technique for Three-Point Second-Order Integrodifferential Boundary Value Problems with-Laplacian

Boundary Value Problems volume 2007, Article number: 057481 (2007) Cite this article

Abstract

A monotone iterative technique is applied to prove the existence of the extremal positive pseudosymmetric solutions for a three-point second-order-Laplacian integrodifferential boundary value problem.

[1234567891011121314151617181920212223242526272829]

References

  1. Il'in VA, Moiseev EI: Nonlocal boundary value problem of the first kind for a Sturm-Liouville operator in its differential and finite difference aspects. Differential Equations 1987,23(7):803-811.

    MATH  MathSciNet  Google Scholar 

  2. Il'in VA, Moiseev EI: Nonlocal boundary-value problem of the secod kind for a Sturm-Liouville operator. Differential Equations 1987,23(8):979-987.

    MATH  MathSciNet  Google Scholar 

  3. Gupta CP: Solvability of a three-point nonlinear boundary value problem for a second order ordinary differential equation. Journal of Mathematical Analysis and Applications 1992,168(2):540-551. 10.1016/0022-247X(92)90179-H

    Article  MATH  MathSciNet  Google Scholar 

  4. Lian WC, Wong FH, Yeh CC: On the existence of positive solutions of nonlinear second order differential equations. Proceedings of the American Mathematical Society 1996,124(4):1117-1126. 10.1090/S0002-9939-96-03403-X

    Article  MATH  MathSciNet  Google Scholar 

  5. Ma R: Positive solutions of a nonlinear three-point boundary-value problem. Electronic Journal of Differential Equations 1999, (34):1-8.

    Google Scholar 

  6. Ma R, Castaneda N:Existence of solutions of nonlinear-point boundary-value problems. Journal of Mathematical Analysis and Applications 2001,256(2):556-567. 10.1006/jmaa.2000.7320

    Article  MATH  MathSciNet  Google Scholar 

  7. Coppel WA: Disconjugacy, Lecture Notes in Mathematics. Volume 220. Springer, New York, NY, USA; 1971:iv+148.

    Google Scholar 

  8. Eloe PW, Ahmad B:Positive solutions of a nonlinearth order boundary value problem with nonlocal conditions. Applied Mathematics Letters 2005,18(5):521-527. 10.1016/j.aml.2004.05.009

    Article  MATH  MathSciNet  Google Scholar 

  9. Wang J-Y, Zheng D-W:On the existence of positive solutions to a three-point boundary value problem for the one-dimensional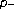Laplacian. Zeitschrift für Angewandte Mathematik und Mechanik 1997,77(6):477-479. 10.1002/zamm.19970770618

    Article  MATH  Google Scholar 

  10. He X, Ge W:A remark on some three-point boundary value problems for the one-dimensionalLaplacian. Zeitschrift für Angewandte Mathematik und Mechanik 2002,82(10):728-731. 10.1002/1521-4001(200210)82:10<728::AID-ZAMM728>3.0.CO;2-R

    Article  MATH  MathSciNet  Google Scholar 

  11. Avery R, Henderson J:Existence of three positive pseudo-symmetric solutions for a one-dimensionalLaplacian. Journal of Mathematical Analysis and Applications 2003,277(2):395-404. 10.1016/S0022-247X(02)00308-6

    Article  MATH  MathSciNet  Google Scholar 

  12. Guo Y, Ge W:Three positive solutions for the one-dimensional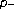Laplacian. Journal of Mathematical Analysis and Applications 2003,286(2):491-508. 10.1016/S0022-247X(03)00476-1

    Article  MATH  MathSciNet  Google Scholar 

  13. He X, Ge W:Twin positive solutions for the one-dimensional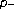Laplacian boundary value problems. Nonlinear Analysis 2004,56(7):975-984. 10.1016/j.na.2003.07.022

    Article  MATH  MathSciNet  Google Scholar 

  14. Li J, Shen J:Existence of three positive solutions for boundary value problems with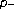Laplacian. Journal of Mathematical Analysis and Applications 2005,311(2):457-465. 10.1016/j.jmaa.2005.02.054

    Article  MATH  MathSciNet  Google Scholar 

  15. Wang Z, Zhang J:Positive solutions for one-dimensionalLaplacian boundary value problems with dependence on the first order derivative. Journal of Mathematical Analysis and Applications 2006,314(2):618-630. 10.1016/j.jmaa.2005.04.012

    Article  MATH  MathSciNet  Google Scholar 

  16. Wang Y, Hou C:Existence of multiple positive solutions for one-dimensionalLaplacian. Journal of Mathematical Analysis and Applications 2006,315(1):144-153. 10.1016/j.jmaa.2005.09.085

    Article  MATH  MathSciNet  Google Scholar 

  17. Ma D-X, Du Z-J, Ge W-G:Existence and iteration of monotone positive solutions for multipoint boundary value problem withLaplacian operator. Computers & Mathematics with Applications 2005,50(5-6):729-739. 10.1016/j.camwa.2005.04.016

    Article  MATH  MathSciNet  Google Scholar 

  18. Ma D-X, Ge W:Existence and iteration of positive pseudo-symmetric solutions for a three-point second orderLaplacian BVP. Applied Mathematics Letters 2007.

    Google Scholar 

  19. Amann H: Fixed point equations and nonlinear eigenvalue problems in ordered Banach spaces. SIAM Review 1976,18(4):620-709. 10.1137/1018114

    Article  MATH  MathSciNet  Google Scholar 

  20. Ladde GS, Lakshmikantham V, Vatsala AS: Monotone Iterative Techniques for Nonlinear Differential Equations, Monographs, Advanced Texts and Surveys in Pure and Applied Mathematics. Volume 27. Pitman, Boston, Mass, USA; 1985:x+236.

    Google Scholar 

  21. Nieto JJ, Jiang Y, Jurang Y: Monotone iterative method for functional-differential equations. Nonlinear Analysis 1998,32(6):741-747. 10.1016/S0362-546X(97)00524-5

    Article  MATH  MathSciNet  Google Scholar 

  22. Vatsala AS, Yang J: Monotone iterative technique for semilinear elliptic systems. Boundary Value Problems 2005,2005(2):93-106. 10.1155/BVP.2005.93

    Article  MATH  MathSciNet  Google Scholar 

  23. Drici Z, McRae FA, Vasundhara Devi J: Monotone iterative technique for periodic boundary value problems with causal operators. Nonlinear Analysis 2006,64(6):1271-1277. 10.1016/j.na.2005.06.033

    Article  MATH  MathSciNet  Google Scholar 

  24. West IH, Vatsala AS: Generalized monotone iterative method for initial value problems. Applied Mathematics Letters 2004,17(11):1231-1237. 10.1016/j.aml.2004.03.003

    Article  MATH  MathSciNet  Google Scholar 

  25. Jiang D, Nieto JJ, Zuo W: On monotone method for first and second order periodic boundary value problems and periodic solutions of functional differential equations. Journal of Mathematical Analysis and Applications 2004,289(2):691-699. 10.1016/j.jmaa.2003.09.020

    Article  MATH  MathSciNet  Google Scholar 

  26. Nieto JJ, Rodríguez-López R: Monotone method for first-order functional differential equations. Computers & Mathematics with Applications 2006,52(3-4):471-484. 10.1016/j.camwa.2006.01.012

    Article  MATH  MathSciNet  Google Scholar 

  27. Ahmad B, Sivasundaram S: The monotone iterative technique for impulsive hybrid set valued integro-differential equations. Nonlinear Analysis 2006,65(12):2260-2276. 10.1016/j.na.2006.01.033

    Article  MATH  MathSciNet  Google Scholar 

  28. Nieto JJ: An abstract monotone iterative technique. Nonlinear Analysis 1997,28(12):1923-1933. 10.1016/S0362-546X(97)89710-6

    Article  MATH  MathSciNet  Google Scholar 

  29. Liz E, Nieto JJ: An abstract monotone iterative method and applications. Dynamic Systems and Applications 1998,7(3):365-375.

    MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Science, King Abdulaziz University, P.O. Box 80203, Jeddah, 21589, Saudi Arabia

    Bashir Ahmad

  2. Departamento de Análisis Matemático, Facultad de Matemáticas, Universidad de Santiago de Compostela, Santiago de Compostela, 15782, Spain

    Juan J Nieto

Authors
  1. Bashir Ahmad
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Juan J Nieto
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Bashir Ahmad.

Rights and permissions

Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

Reprints and Permissions

About this article

Cite this article

Ahmad, B., Nieto, J.J. The Monotone Iterative Technique for Three-Point Second-Order Integrodifferential Boundary Value Problems with-Laplacian. Bound Value Probl 2007, 057481 (2007). https://doi.org/10.1155/2007/57481

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1155/2007/57481

Keywords