Skip to main content
Boundary Value Problems
Download PDF

Parabolic inequalities with nonstandard growths and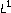 data

Boundary Value Problems volume 2006, Article number: 29286 (2006) Cite this article

Abstract

We prove an existence result for solutions of nonlinear parabolic inequalities with data in Orlicz spaces.

[1234567891011121314]

References

  1. Adams RA: Sobolev Spaces, Pure and Applied Mathematics. Volume 65. Academic Press, New York; 1975:xviii+268.

    Google Scholar 

  2. Donaldson T: Inhomogeneous Orlicz-Sobolev spaces and nonlinear parabolic initial value problems. Journal of Differential Equations 1974,16(2):201-256. 10.1016/0022-0396(74)90012-6

    Article  MathSciNet  MATH  Google Scholar 

  3. Elmahi A, Meskine D: Parabolic equations in Orlicz spaces. Journal of the London Mathematical Society. Second Series 2005,72(2):410-428. 10.1112/S0024610705006630

    Article  MathSciNet  MATH  Google Scholar 

  4. Elmahi A, Meskine D:Strongly nonlinear parabolic equations with natural growth terms and data in Orlicz spaces. Portugaliae Mathematica. Nova Série 2005,62(2):143-183.

    MathSciNet  MATH  Google Scholar 

  5. Elmahi A, Meskine D: Strongly nonlinear parabolic equations with natural growth terms in Orlicz spaces. Nonlinear Analysis. Theory, Methods & Applications 2005,60(1):1-35.

    Article  MathSciNet  MATH  Google Scholar 

  6. Gossez J-P: Nonlinear elliptic boundary value problems for equations with rapidly (or slowly) increasing coefficients. Transactions of the American Mathematical Society 1974, 190: 163-205.

    Article  MathSciNet  MATH  Google Scholar 

  7. Gossez J-P: Some approximation properties in Orlicz-Sobolev spaces. Studia Mathematica 1982,74(1):17-24.

    MathSciNet  MATH  Google Scholar 

  8. Gossez J-P: A strongly nonlinear elliptic problem in Orlicz-Sobolev spaces. In Nonlinear Functional Analysis and Its Applications, Part 1 (Berkeley, Calif, 1983), Proc. Sympos. Pure Math.. Volume 45. American Mathematical Society, Rhode Island; 1986:455-462.

    Chapter  Google Scholar 

  9. Gossez J-P, Mustonen V: Variational inequalities in Orlicz-Sobolev spaces. Nonlinear Analysis. Theory, Methods & Applications 1987,11(3):379-392. 10.1016/0362-546X(87)90053-8

    Article  MathSciNet  MATH  Google Scholar 

  10. Le VK, Schmitt K: Quasilinear elliptic equations and inequalities with rapidly growing coefficients. Journal of the London Mathematical Society. Second Series 2000,62(3):852-872. 10.1112/S0024610700001423

    Article  MathSciNet  MATH  Google Scholar 

  11. Robert J: Inéquations variationnelles paraboliques fortement non linéaires. Journal de Mathématiques Pures et Appliquées. Neuvième Série 1974, 53: 299-320.

    MATH  Google Scholar 

  12. Rudd M: Nonlinear constrained evolution in Banach spaces, M.S. thesis. University of Utah, Utah; 2003.

    Google Scholar 

  13. Rudd M: Weak and strong solvability of parabolic variational inequalities in Banach spaces. Journal of Evolution Equations 2004,4(4):497-517. 10.1007/s00028-004-0153-z

    Article  MathSciNet  MATH  Google Scholar 

  14. Rudd M, Schmitt K: Variational inequalities of elliptic and parabolic type. Taiwanese Journal of Mathematics 2002,6(3):287-322.

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. LERMA, École Mohammadia d'Ingénieurs, Université Mahammed V-Agdal, Avenue Ibn Sina, Rabat-Agdal, BP 765, Morocco

    R Aboulaich, B Achchab, D Meskine & A Souissi

  2. Faculté des Sciences Juridiques, Économiques et Sociales, Université Hassan 1er, Settat, BP 784, Morocco

    B Achchab

  3. GAN, Département de Mathématiques et d'Informatiques, Faculté des Sciences, Université Mahammed V-Agdal, Avenue Ibn Battouta, Rabat, BP 1014, Morocco

    D Meskine & A Souissi

Authors
  1. R Aboulaich
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. B Achchab
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. D Meskine
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. A Souissi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to D Meskine.

Rights and permissions

Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License ( https://creativecommons.org/licenses/by/2.0 ), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

Reprints and permissions

About this article

Cite this article

Aboulaich, R., Achchab, B., Meskine, D. et al. Parabolic inequalities with nonstandard growths and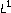 data. Bound Value Probl 2006, 29286 (2006). https://doi.org/10.1155/BVP/2006/29286

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1155/BVP/2006/29286

Keywords