- Research Article
- Open access
- Published:
Existence of Solutions for the 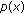 -Laplacian Problem with Singular Term
-Laplacian Problem with Singular Term
Boundary Value Problems volume 2010, Article number: 584843 (2010)
Abstract
We study the following  -Laplacian problem with singular term:
-Laplacian problem with singular term:  ,
,  ,
,  ,
,  , where
, where  is a bounded domain,
is a bounded domain,  . We obtain the existence of solutions in
. We obtain the existence of solutions in  .
.
1. Introduction
After Kováčik and Rákosník first discussed the  spaces and
spaces and  spaces in [1], a lot of research has been done concerning these kinds of variable exponent spaces, for example, see [2–5] for the properties of such spaces and [6–9] for the applications of variable exponent spaces on partial differential equations. Especially in
spaces in [1], a lot of research has been done concerning these kinds of variable exponent spaces, for example, see [2–5] for the properties of such spaces and [6–9] for the applications of variable exponent spaces on partial differential equations. Especially in  spaces, there are a lot of studies on
spaces, there are a lot of studies on  -Laplacian problems; see [8, 9]. In the recent years, the theory of problems with
-Laplacian problems; see [8, 9]. In the recent years, the theory of problems with  -growth conditions has important applications in nonlinear elastic mechanics and electrorheological fluids (see [10–14]).
-growth conditions has important applications in nonlinear elastic mechanics and electrorheological fluids (see [10–14]).
In this paper, we study the existence of the weak solutions for the following  -Laplacian problem:
-Laplacian problem:

where  is a bounded domain,
is a bounded domain,  ,
,  is Lipschitz continuous on
is Lipschitz continuous on  , and
, and 
Let  be the set of all Lebesgue measurable functions
be the set of all Lebesgue measurable functions  . For all
. For all  , we denote
, we denote  ,
,  , and denote by
, and denote by  the fact that
the fact that  .
.
We impose the following condition on  :
:
(F)  ,
,  ,
,  and for
and for  , there exist
, there exist such that
such that  , whenever
, whenever  .
.
A typical example of (1.1) is the following problem involving subcritical Sobolev-Hardy exponents of the form

where  ,
,  ,
,  ,
,  . In fact, take
. In fact, take  ,
,  , and
, and  , then it is easy to verify that (F) is satisfied.
, then it is easy to verify that (F) is satisfied.
Our object is to obtain the existence of solutions in the following four cases:
(1)  ;
;
(2)  ;
;
(3)  ;
;
(4)  .
.
When  , the solution of the
, the solution of the  -Laplacian equations without singularity has been studied by many researchers. The study of problem (1.1) with variable exponents is a new topic.
-Laplacian equations without singularity has been studied by many researchers. The study of problem (1.1) with variable exponents is a new topic.
The paper is organized as follows. In Section 2, we present some necessary preliminary knowledge of variable exponent Lebesgue and Sobolev spaces. In Section 3, we prove our main results.
2. Preliminaries
In this section we first recall some facts on variable exponent Lebesgue space  and variable exponent Sobolev space
and variable exponent Sobolev space  , where
, where  is an open set; see [1–4, 8, 15] for the details.
is an open set; see [1–4, 8, 15] for the details.
Let  and
and

The variable exponent Lebesgue space  is the class of functions
is the class of functions  such that
such that  .
.  is a Banach space endowed with the norm (2.1).
is a Banach space endowed with the norm (2.1).
For a given  we define the conjugate function
we define the conjugate function  as
as

Theorem 2.1.
Let  . Then the inequality
. Then the inequality

holds for every  and
and  with the constant
with the constant  depending on
depending on  and
and  only.
only.
Theorem 2.2.
The dual space of  is
is  if and only if
if and only if  . The space
. The space  is reflexive if and only if
is reflexive if and only if

Theorem 2.3.
Suppose that  satisfies (2.4). Let
satisfies (2.4). Let  ,
,  , then necessary and sufficient condition for
, then necessary and sufficient condition for  is that for almost all
is that for almost all  one has
one has  , and in this case, the imbedding is continuous.
, and in this case, the imbedding is continuous.
Theorem 2.4.
Suppose that  satisfies (2.4). Let
satisfies (2.4). Let  . If
. If  , then
, then
(1)  if and only if
if and only if  ,
,
(2) if  , then
, then  ,
,
(3) if  , then
, then  ,
,
(4)  if and only if
if and only if  ,
,
(5)  if and only if
if and only if  .
.
We assume that  is a given positive integer.
is a given positive integer.
Given a multi-index  , we set
, we set  and
and  , where
, where  is the generalized derivative operator.
is the generalized derivative operator.
The generalized Sobolev space  is the class of functions
is the class of functions  on
on  such that
such that  for every multi-index
for every multi-index  with
with  , endowed with the norm
, endowed with the norm

By  we denote the subspace of
we denote the subspace of  which is the closure of
which is the closure of  with respect to the norm (2.5).
with respect to the norm (2.5).
In this paper we use the following equivalent norm on  :
:

Then we have the inequality  .
.
Theorem 2.5.
The spaces  and
and  are separable reflexive Banach spaces, if
are separable reflexive Banach spaces, if  satisfies (2.4).
satisfies (2.4).
Theorem 2.6.
Suppose that  satisfies (2.4). Let
satisfies (2.4). Let  . If
. If  , then
, then
(1)  if and only if
if and only if  ,
,
(2) if  , then
, then  ,
,
(3) if  , then
, then  ,
,
(4)  if and only if
if and only if  ,
,
(5)  if and only if
if and only if  .
.
We denote the dual space of  by
by  , then we have the following.
, then we have the following.
Theorem 2.7.
Let  . Then for every
. Then for every  there exists a unique system of functions
there exists a unique system of functions  such that
such that

The norm of  is defined as
is defined as

Theorem 2.8.
Let  be a domain in
be a domain in  with cone property. If
with cone property. If  is Lipschitz continuous and
is Lipschitz continuous and  ,
,  is measurable and satisfies
is measurable and satisfies  a.e.
a.e.  , then there is a continuous embedding
, then there is a continuous embedding  .
.
Theorem 2.9.
Let  be a bounded domain. If
be a bounded domain. If  and
and  , then
, then

where  is a constant depending on
is a constant depending on  .
.
Next let us consider the weighted variable exponent Lebesgue space. Let  , and
, and  for
for  . Define
. Define

with the norm

then  is a Banach space.
is a Banach space.
Theorem 2.10.
Suppose that  satisfies (2.4). Let
satisfies (2.4). Let  . If
. If  ,
, , then
, then
(1) for  ,
,  if and only if
if and only if  ,
,
(2)  if and only if
if and only if  ,
,
(3) if  , then
, then  ,
,
(4) if  , then
, then  ,
,
(5)  if and only if
if and only if  ,
,
(6)  if and only if
if and only if  .
.
Theorem 2.11.
Assume that the boundary of  possesses the cone property and
possesses the cone property and  . Suppose that
. Suppose that  , and
, and  , for
, for  . If
. If  and
and  then there is a compact embedding
then there is a compact embedding  .
.
Theorem 2.12.
Let  be a measurable subset. Let
be a measurable subset. Let  be a Caracheodory function and satisfies
be a Caracheodory function and satisfies

where  ,
,  ,
,  ,
,  is a constant, then the Nemytsky operator from
is a constant, then the Nemytsky operator from  to
to  defined by
defined by  is a continuous and bounded operator.
is a continuous and bounded operator.
3. Existence and Multiplicity of Solutions
Let

The critical points of  , that is,
, that is,

for all  , are weak solutions of problem (1.1). So we need only to consider the existence of nontrivial critical points of
, are weak solutions of problem (1.1). So we need only to consider the existence of nontrivial critical points of  .
.
Denote by  , and
, and  the generic positive constants. Denote by
the generic positive constants. Denote by  the Lebesgue measure of
the Lebesgue measure of  .
.
To study the existence of solutions for problem (1.1) in the first case, we additionally impose the following conditions.
(A-1)  and
and  ,
,  .
.
(B-1) There exists a function  , such that
, such that  and
and  for
for  ,
,  .
.
(C-1) there exist such that
such that  for
for  ,
,  , where
, where  and
and  .
.
(D-1)  , for all
, for all  .
.
Theorem 3.1.
Under assumptions (F) and (A-1)–(C-1), problem (1.1) admits a nontrivial solution.
Proof.
First we show that any  sequence is bounded. Let
sequence is bounded. Let  and
and  , such that
, such that  and
and  in
in  . By (A-1) and (B-1),
. By (A-1) and (B-1),  , and
, and  . Let
. Let  and
and  , then
, then  ,
,  ,
,  ,
,  and
and  . Let
. Let  and
and  . Then
. Then

By (B-1), we get

By (F), we get  , so there exist
, so there exist such that
such that  on
on  . Note
. Note  , so we have
, so we have

By Young's inequality, for  , we get
, we get

Take  sufficiently small so that
sufficiently small so that  .
.
Note that  , by Young's inequality again and for
, by Young's inequality again and for  , we get
, we get

Take  sufficiently small so that
sufficiently small so that  .
.
From the above remark, we have

As  ,
,  and
and  , we have
, we have  . Since
. Since  and
and  , by Theorem 2.6 we have
, by Theorem 2.6 we have

when  and
and  is sufficiently large. Then it is easy to see that
is sufficiently large. Then it is easy to see that  is bounded in
is bounded in  . Next we show that
. Next we show that  possesses a convergent subsequence (still denoted by
possesses a convergent subsequence (still denoted by  ).
).
Note that

Because  is bounded in
is bounded in  , there exists a subsequence
, there exists a subsequence  (still denoted by
(still denoted by  ), such that
), such that  weakly in
weakly in  . By Theorem 2.11, there are compact embeddings
. By Theorem 2.11, there are compact embeddings  and
and  , then
, then  in
in  and
and  . So we get
. So we get

Hence  as
as  .
.
By (F), we have

and similarly for every  ,
,

Since

and  in
in  and
and  , we obtain
, we obtain  and
and  . Similarly,
. Similarly,

Because  is bounded, we get
is bounded, we get  , as
, as  . From the above remark, we conclude
. From the above remark, we conclude  , as
, as  .
.
Thus  , as
, as  . Then we get
. Then we get  . As in the proof of Theorem
. As in the proof of Theorem  in [6, 7], we divide
in [6, 7], we divide  into the following two parts:
into the following two parts:

On  , we have
, we have

Then  as
as  .
.
On  , we have
, we have

so  , as
, as  .
.
Thus we get  . Then
. Then  in
in  .
.
From (F) and (B-1) we have  , for all
, for all  ,
,  . So we get
. So we get  , for all
, for all  ,
,  . for all
. for all  , take
, take  , then
, then  . Since
. Since  and
and  , there exists
, there exists  such that
such that  , and
, and  , for
, for  . Let
. Let  , such that
, such that  for
for  ,
,  for
for  , and
, and  in
in  . Then we have
. Then we have

where  . So if
. So if  is sufficiently large, we obtain
is sufficiently large, we obtain  .
.
From (F) and (C-1), we have  , then
, then  . So we get
. So we get

Let  , then
, then  . By Theorem 2.11,
. By Theorem 2.11,  ,
,  and
and  . When
. When  is sufficiently small,
is sufficiently small,  ,
,  and
and  . For any
. For any  , as
, as  , for any
, for any  , we can find
, we can find  such that
such that  and
and  whenever
whenever  . Take
. Take  , then
, then  .
.  is an open covering of
is an open covering of  . As
. As  is compact, we can pick a finite subcovering
is compact, we can pick a finite subcovering  for
for  from the covering
from the covering  . If
. If  we define
we define  on
on  . We can use all the hyperplanes, for each of which there exists at least one hypersurface of some
. We can use all the hyperplanes, for each of which there exists at least one hypersurface of some  lying on it, to divide
lying on it, to divide  into finite open hypercubes
into finite open hypercubes  which mutually have no common points. It is obvious that
which mutually have no common points. It is obvious that  and for each
and for each  there exists at least one
there exists at least one  such that
such that  . Let
. Let  , then
, then  and we have
and we have

If  is sufficiently small such that
is sufficiently small such that

we have  .
.
The mountain pass theorem guarantees that  has a nontrivial critical point
has a nontrivial critical point  .
.
Since  is a separable and reflexive Banach space, there exist
is a separable and reflexive Banach space, there exist  and
and  such that
such that

For  , denote
, denote  ,
,  ,
,  .
.
Theorem 3.2.
Under assumptions (F), (A-1)–(D-1), problem (1.1) admits a sequence of solutions  such that
such that  .
.
Proof.
Let  . We first show that
. We first show that  is weakly strongly continuous. Let
is weakly strongly continuous. Let  weakly in
weakly in  . By the compact embedding
. By the compact embedding  , we have
, we have  and
and  a.e. on
a.e. on  . By the inequality
. By the inequality  and the Vitali Theorem, we get
and the Vitali Theorem, we get  .
.
Note that

When  ,
,

When  ,
,  is bounded. So we get
is bounded. So we get

By the compact embedding  , we get
, we get  in
in  . So by Theorem 2.12 we obtain
. So by Theorem 2.12 we obtain  , that is,
, that is,  . Hence we obtain that
. Hence we obtain that  is weakly strongly continuous. By Proposition
is weakly strongly continuous. By Proposition  in [8],
in [8],  as
as  for
for  . For all
. For all  , there exists a positive integer
, there exists a positive integer  such that
such that  for all
for all  . Assume
. Assume  for each
for each  . Define
. Define  in the following way:
in the following way:

Note that  as
as  . Hence for
. Hence for  with
with  , we get
, we get

So

Note that  and
and  . Since the dimension of
. Since the dimension of  is finite, any two norms on
is finite, any two norms on  are equivalent, then
are equivalent, then  . If
. If  , it is immediate that
, it is immediate that  . If
. If  , then
, then  . As in the proof of Theorem 3.1 we can find hypercubes
. As in the proof of Theorem 3.1 we can find hypercubes  which mutually have no common points such that
which mutually have no common points such that  and
and  , where
, where  . Then we need only to consider the case:
. Then we need only to consider the case:  for every
for every  . We have
. We have

Let  ,
,  . Let
. Let  and
and  . Denote
. Denote  . Let
. Let  be sufficiently large such that
be sufficiently large such that  . There at least exists one
. There at least exists one  such that
such that  . We have
. We have

and  as
as  . Hence we obtain that
. Hence we obtain that  as
as  . Thus for each
. Thus for each  , there exists
, there exists  such that
such that  for
for  . From Theorem 3.1
. From Theorem 3.1  satisfies
satisfies  condition. In view of (D-1), by Fountain Theorem (see [16]), we conclude the result.
condition. In view of (D-1), by Fountain Theorem (see [16]), we conclude the result.
In the second case, we additionally impose the following condition:
(A-2)  and
and  .
.
Theorem 3.3.
Under assumptions (F), (A-2), (B-1), and (C-1) there exist  such that when
such that when  , problem (1.1) admits a nontrivial solution.
, problem (1.1) admits a nontrivial solution.
Proof.
It is obvious that  . Let
. Let  be such that
be such that  . Since
. Since  , there exists
, there exists  and
and  such that
such that  , for all
, for all  . Thus
. Thus  for all
for all  . Let
. Let  be as defined in Theorem 3.1. By (C-1),
be as defined in Theorem 3.1. By (C-1),  , and
, and  , when
, when  . Then for any
. Then for any  and
and  , we have
, we have

If  is sufficiently small,
is sufficiently small,  .
.
From (F) and (C-1), we have  and
and  . By Theorems 2.8 and 2.11, there exist positive constants
. By Theorems 2.8 and 2.11, there exist positive constants  such that
such that  ,
,  ,
,  . When
. When  is sufficiently small, we have
is sufficiently small, we have  ,
,  , and
, and  . As in the proof of Theorem 3.1 we can find hypercubes
. As in the proof of Theorem 3.1 we can find hypercubes  which mutually have no common points such that
which mutually have no common points such that  ,
,  and
and  , where
, where  . Then
. Then

Since  , for all
, for all  , when
, when  ,
,  . Fix
. Fix  such that
such that  . Then we have
. Then we have

Let  . When
. When  ,
,  . As in the proof of Theorem
. As in the proof of Theorem  in [17], denote
in [17], denote  , we have
, we have  and
and  . Let
. Let  . Applying Ekeland's variational principle to the functional
. Applying Ekeland's variational principle to the functional  , we find
, we find  such that
such that  ,
,  ,
,  , and
, and  . Thus we get a sequence
. Thus we get a sequence  such that
such that  and
and  . It is clear that
. It is clear that  is bounded in
is bounded in  . As in the proof of Theorem 3.1, we get a subsequence of
. As in the proof of Theorem 3.1, we get a subsequence of  , still denoted by
, still denoted by  , such that
, such that  in
in  . So
. So  and
and  .
.
Theorem 3.4.
Under assumptions (F), (A-2), and (B-1)–(D-1), problem (1.1) has a sequence of solutions  such that
such that  .
.
Proof.
First we show that any  sequence is bounded. Let
sequence is bounded. Let  and
and  , such that
, such that  and
and  in
in  . By (B-1), there exist
. By (B-1), there exist such that
such that  . From (F), (A-2), and (B-1)–(D-1), we have
. From (F), (A-2), and (B-1)–(D-1), we have

Thus we have

By Young's inequality, for  , we get
, we get

Take  ,
, and
and  sufficiently small so that
sufficiently small so that  ,
,  and
and  , then
, then

Therefore by Theorems 2.6 and 2.9, we get that  is bounded in
is bounded in  . Then as in the proof of Theorem 3.1
. Then as in the proof of Theorem 3.1 possesses a convergent subsequence
possesses a convergent subsequence  (still denoted by
(still denoted by  ). By Theorem 3.2, we can also have
). By Theorem 3.2, we can also have

As in the proof of Theorem 3.1 we can find hypercubes  which mutually have no common points such that
which mutually have no common points such that  and
and  , where
, where  . Since the dimension of
. Since the dimension of  is finite, any two norms on
is finite, any two norms on  are equivalent. Then we need only to consider the cases
are equivalent. Then we need only to consider the cases  and
and  for every
for every  . We have
. We have

Hence  as
as  . As in the proof of Theorem 3.2, we complete the proof.
. As in the proof of Theorem 3.2, we complete the proof.
In the third case, we additionally impose the following condition:
(A-3)  , and
, and  ,
,
(B-3) there exist  such that
such that  for
for  .
.
Theorem 3.5.
Under assumptions (F), (A-3), and (B-3), problem (1.1) admits a nontrivial solution.
Proof.
By Young's inequality, for  , we get
, we get  . By (F), we have
. By (F), we have  . Thus
. Thus

Take  sufficiently small so that
sufficiently small so that  . From Theorem 2.11,
. From Theorem 2.11,  . If
. If  ,
,  is bounded. Then we need only to consider the case
is bounded. Then we need only to consider the case  . As in the proof of Theorem 3.1 we can find hypercubes
. As in the proof of Theorem 3.1 we can find hypercubes  which mutually have no common points such that
which mutually have no common points such that  and
and  , where
, where  . Then we have
. Then we have

where  , and
, and  ,
,  . As in the proof of Theorem 3.2, we obtain that
. As in the proof of Theorem 3.2, we obtain that  is coercive, that is,
is coercive, that is,  as
as  . Thus
. Thus  has a critical point
has a critical point  such that
such that  and further
and further  is a weak solution of (1.1).
is a weak solution of (1.1).
Next we show that  is nontrivial. Let
is nontrivial. Let  be the same as that in Theorem 3.3. By (B-3),
be the same as that in Theorem 3.3. By (B-3),  . Then
. Then

If  is sufficiently small,
is sufficiently small,  .
.
In the fourth case, we additionally impose the following condition:
(A-4)  and
and  .
.
Theorem 3.6.
Under assumptions (F), (A-4), and (D-1), problem (1.1) admits a sequence of solutions  such that
such that  .
.
Proof.
First we show that any  sequence is bounded. Let
sequence is bounded. Let  and
and  , such that
, such that  and
and  in
in  . Denote
. Denote  and
and  . We have
. We have  and
and  .
.
We can get

By Young's inequality, for  , we get
, we get

Take  and
and  sufficiently small so that
sufficiently small so that  and
and  . Then
. Then

As in the proof of Theorem 3.5,  , when
, when  . Thus, we conclude that
. Thus, we conclude that  is bounded in
is bounded in  . Then as in the proof of Theorem 3.1
. Then as in the proof of Theorem 3.1 possesses a convergent subsequence
possesses a convergent subsequence  (still denoted by
(still denoted by  ). By Theorem 3.2, we can also get
). By Theorem 3.2, we can also get

As in the proof of Theorem 3.1 we can find hypercubes  which mutually have no common points such that
which mutually have no common points such that  and
and  , where
, where  . Since the dimension of
. Since the dimension of  is finite, any two norms on
is finite, any two norms on  are equivalent. Then we need only to consider the cases
are equivalent. Then we need only to consider the cases 
 and
and  for every
for every  . We have
. We have

Hence we obtain  as
as  . As in the proof of Theorem 3.2, we complete the proof.
. As in the proof of Theorem 3.2, we complete the proof.
References
Kováčik O, Rákosník J:On spaces
 and
and 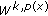 . Czechoslovak Mathematical Journal 1991,41(4):592-618.
. Czechoslovak Mathematical Journal 1991,41(4):592-618.Edmunds D, Lang J, Nekvinda A:On
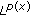 norms. Proceedings of The Royal Society of London. Series A 1999,455(1981):219-225. 10.1098/rspa.1999.0309
norms. Proceedings of The Royal Society of London. Series A 1999,455(1981):219-225. 10.1098/rspa.1999.0309Edmunds D, Rákosník J: Sobolev embeddings with variable exponent. Studia Mathematica 2000,143(3):267-293.
Fan X, Shen J, Zhao D:Sobolev embedding theorems for spaces
 . Journal of Mathematical Analysis and Applications 2001,262(2):749-760. 10.1006/jmaa.2001.7618
. Journal of Mathematical Analysis and Applications 2001,262(2):749-760. 10.1006/jmaa.2001.7618Fan X, Zhang Q, Zhao D:Eigenvalues of
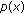 -Laplacian Dirichlet problem. Journal of Mathematical Analysis and Applications 2005,302(2):306-317. 10.1016/j.jmaa.2003.11.020
-Laplacian Dirichlet problem. Journal of Mathematical Analysis and Applications 2005,302(2):306-317. 10.1016/j.jmaa.2003.11.020Chabrowski J, Fu Y:Existence of solutions for
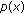 -Laplacian problems on a bounded domain. Journal of Mathematical Analysis and Applications 2005,306(2):604-618. 10.1016/j.jmaa.2004.10.028
-Laplacian problems on a bounded domain. Journal of Mathematical Analysis and Applications 2005,306(2):604-618. 10.1016/j.jmaa.2004.10.028Chabrowski J, Fu Y:Corrigendum to "Existence of solutions for
 -Laplacian problems on a bounded domain".
-Laplacian problems on a bounded domain".Fan X:Solutions for
 -Laplacian dirichlet problems with singular coefficients. Journal of Mathematical Analysis and Applications 2005,312(2):464-477. 10.1016/j.jmaa.2005.03.057
-Laplacian dirichlet problems with singular coefficients. Journal of Mathematical Analysis and Applications 2005,312(2):464-477. 10.1016/j.jmaa.2005.03.057Galewski M:New variational method for the
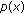 -Laplacian equation. Bulletin of the Australian Mathematical Society 2005,72(1):53-65. 10.1017/S0004972700034870
-Laplacian equation. Bulletin of the Australian Mathematical Society 2005,72(1):53-65. 10.1017/S0004972700034870Acerbi E, Mingione G: Regularity results for stationary electro-rheological fluids. Archive for Rational Mechanics and Analysis 2002,164(3):213-259. 10.1007/s00205-002-0208-7
Acerbi E, Mingione G, Seregin GA: Regularity results for parabolic systems related to a class of non-newtonian fluids. Annales de l'Institut Henri Poincaré. Analyse Non Linéaire 2004,21(1):25-60.
Mihăilescu M, Rădulescu V: A multiplicity result for a nonlinear degenerate problem arising in the theory of electrorheological fluids. Proceedings of the Royal Society of London. Series A 2006,462(2073):2625-2641. 10.1098/rspa.2005.1633
Růžička M: Electrorheological Fluids: Modeling and Mathematical Theory. Springer, Berlin, Germany; 2000.
Zhikov V: Averaging of functionals in the calculus of variations and elasticity. Mathematics of the USSR-Izvestiya 1987, 29: 33-66. 10.1070/IM1987v029n01ABEH000958
Diening L:Riesz potential and Sobolev embeddings on generalized Lebesgue and Sobolev spaces
 and
and 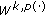 . Mathematische Nachrichten 2004, 268: 31-43. 10.1002/mana.200310157
. Mathematische Nachrichten 2004, 268: 31-43. 10.1002/mana.200310157Willem M: Minimax Theorems, Progress in Nonlinear Differential Equations and Their Applications, 24. Birkhäuser, Boston, Mass, USA; 1996:x+162.
Mihăilescu M, Rădulescu V: On a nonhomogeneous quasilinear eigenvalue problem in Sobolev spaces with variable exponent. Proceedings of the American Mathematical Society 2007,135(9):2929-2937. 10.1090/S0002-9939-07-08815-6
Acknowledgments
This work is supported by the Science Research Foundation in Harbin Institute of Technology (HITC200702), The Natural Science Foundation of Heilongjiang Province (A2007-04), and the Program of Excellent Team in Harbin Institute of Technology.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Yongqiang, F., Mei, Y. Existence of Solutions for the 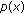 -Laplacian Problem with Singular Term.
Bound Value Probl 2010, 584843 (2010). https://doi.org/10.1155/2010/584843
-Laplacian Problem with Singular Term.
Bound Value Probl 2010, 584843 (2010). https://doi.org/10.1155/2010/584843
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2010/584843
 and
and 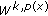 . Czechoslovak Mathematical Journal 1991,41(4):592-618.
. Czechoslovak Mathematical Journal 1991,41(4):592-618.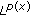 norms. Proceedings of The Royal Society of London. Series A 1999,455(1981):219-225. 10.1098/rspa.1999.0309
norms. Proceedings of The Royal Society of London. Series A 1999,455(1981):219-225. 10.1098/rspa.1999.0309 . Journal of Mathematical Analysis and Applications 2001,262(2):749-760. 10.1006/jmaa.2001.7618
. Journal of Mathematical Analysis and Applications 2001,262(2):749-760. 10.1006/jmaa.2001.7618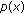 -Laplacian Dirichlet problem. Journal of Mathematical Analysis and Applications 2005,302(2):306-317. 10.1016/j.jmaa.2003.11.020
-Laplacian Dirichlet problem. Journal of Mathematical Analysis and Applications 2005,302(2):306-317. 10.1016/j.jmaa.2003.11.020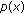 -Laplacian problems on a bounded domain. Journal of Mathematical Analysis and Applications 2005,306(2):604-618. 10.1016/j.jmaa.2004.10.028
-Laplacian problems on a bounded domain. Journal of Mathematical Analysis and Applications 2005,306(2):604-618. 10.1016/j.jmaa.2004.10.028 -Laplacian problems on a bounded domain".
-Laplacian problems on a bounded domain". -Laplacian dirichlet problems with singular coefficients. Journal of Mathematical Analysis and Applications 2005,312(2):464-477. 10.1016/j.jmaa.2005.03.057
-Laplacian dirichlet problems with singular coefficients. Journal of Mathematical Analysis and Applications 2005,312(2):464-477. 10.1016/j.jmaa.2005.03.057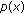 -Laplacian equation. Bulletin of the Australian Mathematical Society 2005,72(1):53-65. 10.1017/S0004972700034870
-Laplacian equation. Bulletin of the Australian Mathematical Society 2005,72(1):53-65. 10.1017/S0004972700034870 and
and 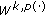 . Mathematische Nachrichten 2004, 268: 31-43. 10.1002/mana.200310157
. Mathematische Nachrichten 2004, 268: 31-43. 10.1002/mana.200310157