Skip to main content
Boundary Value Problems
Download PDF
  • Research Article
  • Open access
  • Published:

Second-order estimates for boundary blowup solutions of special elliptic equations

Boundary Value Problems volume 2006, Article number: 45859 (2006) Cite this article

Abstract

We find a second-order approximation of the boundary blowup solution of the equation, with, in a bounded smooth domain. Furthermore, we consider the equation. In both cases, we underline the effect of the geometry of the domain in the asymptotic expansion of the solutions near the boundary.

[12345678910111213141516]

References

  1. Andersson L, Chruściel PT: Solutions of the constraint equations in general relativity satisfying "hyperboloidal boundary conditions". Dissertationes Mathematicae (Rozprawy Matematyczne) 1996, 355: 1-100.

    MathSciNet  MATH  Google Scholar 

  2. Anedda C, Buttu A, Porru G: Boundary estimates for blow-up solutions of elliptic equations with exponential growth. to appear in Proceedings Differential and Difference Equations

  3. Anedda C, Porru G: Higher order boundary estimates for blow-up solutions of elliptic equations. to appear in Differential Integral Equations

  4. Bandle C: Asymptotic behaviour of large solutions of quasilinear elliptic problems. Zeitschrift für Angewandte Mathematik und Physik 2003,54(5):731-738. 10.1007/s00033-003-3207-0

    Article  MathSciNet  MATH  Google Scholar 

  5. Bandle C, Giarrusso E: Boundary blow up for semilinear elliptic equations with nonlinear gradient terms. Advances in Differential Equations 1996,1(1):133-150.

    MathSciNet  MATH  Google Scholar 

  6. Bandle C, Marcus M: "Large" solutions of semilinear elliptic equations: existence, uniqueness and asymptotic behaviour. Journal d'Analyse Mathématique 1992, 58: 9-24.

    Article  MathSciNet  MATH  Google Scholar 

  7. Bandle C, Marcus M: On second-order effects in the boundary behaviour of large solutions of semilinear elliptic problems. Differential and Integral Equations 1998,11(1):23-34.

    MathSciNet  MATH  Google Scholar 

  8. Bandle C, Marcus M: Dependence of blowup rate of large solutions of semilinear elliptic equations, on the curvature of the boundary. Complex Variables. Theory and Application 2004,49(7–9):555-570.

    Article  MathSciNet  MATH  Google Scholar 

  9. Berhanu S, Porru G: Qualitative and quantitative estimates for large solutions to semilinear equations. Communications in Applied Analysis 2000,4(1):121-131.

    MathSciNet  MATH  Google Scholar 

  10. Bieberbach L: 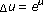und die automorphen Funktionen. Mathematische Annalen 1916,77(2):173-212. 10.1007/BF01456901

    Article  MathSciNet  Google Scholar 

  11. del Pino M, Letelier R: The influence of domain geometry in boundary blow-up elliptic problems. Nonlinear Analysis. Theory, Methods & Applications. Series A: Theory and Methods 2002,48(6):897-904.

    Article  MathSciNet  MATH  Google Scholar 

  12. Gilbarg D, Trudinger NS: Elliptic Partial Differential Equations of Second Order, Grundlehren der mathematischen Wissenschaften. Volume 224. Springer, Berlin; 1977:x+401.

    Book  Google Scholar 

  13. Greco A, Porru G: Asymptotic estimates and convexity of large solutions to semilinear elliptic equations. Differential and Integral Equations 1997,10(2):219-229.

    MathSciNet  MATH  Google Scholar 

  14. Keller JB:On solutions of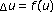. Communications on Pure and Applied Mathematics 1957, 10: 503-510. 10.1002/cpa.3160100402

    Article  MathSciNet  MATH  Google Scholar 

  15. Lazer AC, McKenna PJ: Asymptotic behavior of solutions of boundary blowup problems. Differential and Integral Equations 1994,7(3-4):1001-1019.

    MathSciNet  MATH  Google Scholar 

  16. Osserman R:On the inequality. Pacific Journal of Mathematics 1957,7(4):1641-1647.

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica, Universitá di Cagliari, Via Ospedale 72, Cagliari, 09124, Italy

    Claudia Anedda, Anna Buttu & Giovanni Porru

Authors
  1. Claudia Anedda
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Anna Buttu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Giovanni Porru
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Giovanni Porru.

Rights and permissions

Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License ( https://creativecommons.org/licenses/by/2.0 ), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

Reprints and permissions

About this article

Cite this article

Anedda, C., Buttu, A. & Porru, G. Second-order estimates for boundary blowup solutions of special elliptic equations. Bound Value Probl 2006, 45859 (2006). https://doi.org/10.1155/BVP/2006/45859

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1155/BVP/2006/45859

Keywords